5 Over 7 Septuplets

As you guys may have inferred about me by now, I'm really interested in rhythm. I feel you can add tremendous color and personality to your music through the use of rhythm. Rhythm is as important to me as pitch is to Dan Stearns (although Dan does some very cool things with time, as well).

We are all accustomed to the idea of triplets. Everyone plays them, either intentionally or unintentionally. Triplets are really a form of a polyrhythm in so far as they really represent 3 beats played for every 2 beats counted. Furthermore, triplets are unique in that they involve the use of an odd numbered denominator. What I mean by this is that each beat of, say, an 1/8 note triplet equals 2/3 the beat duration of a "normal" 1/8 note. This certainly makes sense since 3 x 2/3 = 2. Obviously, that's no revelation to any of us. Nevertheless, it does indeed open the door for very interesting applications of this idea.

Now, what is the relationship between tempo and beat duration? Well, that's a manageable question. For instance, let's consider the relationship between a 1/4 note and a 1/2 note. You can look at a 1/4 note as either

Again, this is a very basic thing.

Now suppose we wanted to play 7 beats for every 5 beats. Let's call this a "5 over 7 septuplet". Please keep in mind that I am NOT talking directly about finer subdivisions of a beat. For instance, a lot of Indian music uses finer subdivisions of a beat like septuplets. As an example, a bar of 3/4 using septuplets would be made up of 21 beats per measure. But that is not what is being discussed here (although it can, in some sense, be inferred).

What this really amounts to is counting 5/7ths of an eighth note beat! So if for example, we have an 1/8 note corresponding to 170 BPM, what would be the translated tempo corresponding to these whacked out 5/7th beats? It would just be 7/5 x 170 = 238 BPM. So again, we can look at each beat of these "5 over 7 septuplets" as either 5/7 the beat duration of an 1/8 note or the same 1/8 note played at a tempo 7/5 times the original, in this case 238 BPM.

Now, this would be the prescribed method for developing facility with such a line. You would need to program a drum machine or sequencer click track or whatever option might be available. You need to truncate the 7 beats perfectly within the span of those 5 beats. This is one reason why I chose a tempo of 170. If this 1/8th note tempo is a multiple of 5, which in this case it is, then everything works very cleanly. If not, you would have some "round off error" and subsequent difficulty with the truncation. Again, what we are talking about here is a polyrhythm specific to a small grouping of notes, rather than a bar made up of groupings of notes. It's just a glorified version of a triplet. I mean, why do we restrict ourselves to 1/4 notes, 1/2 notes, 1/8 notes, etc.? Rhythm can certainly be thought of as rational multiples of some tempo. That's all I'm doing here.

So, the music I'm working on these days uses some of these ideas. I've included a quick snippet for you to check out. I wrote this particular piece of music specifically for this "lesson". It's a chord progression based on a Hungarian major scale. The piece is in 5, mostly for the sake of emphasizing the septuplet line. The 5 over 7 septuplet comes in at the beginning of the 5th measure and then again at the top of the 11th measure. In those particular sections I am adding counterpoint with a particular tuba patch to help emphasize the group of 7 notes. In fact, I'm really spelling out the 7 line with straight 5/7 notes. On top of the tuba part is a Sarangi-like line in the 5th measure, followed by an Er-Hu line in the 11th measure. All timbres are generated from an expanded JV1080. The progression is played twice. The second time, however, only has the 2-bar Sarangi 5/7 line. Therefore, the rhythmic contrast will be much clearer and more immediately accessible. It should be easier for you to count the 7 against 5 the second time around. I left the other chords naked for that reason alone. You'll also notice that in both of my 5/7 phrases, I'm playing a triplet figure, as well. So that would actually be (1/2)(2/3)(5/7) = 5/21st of a normal 1/8 note pulse. Try friggin' counting that.

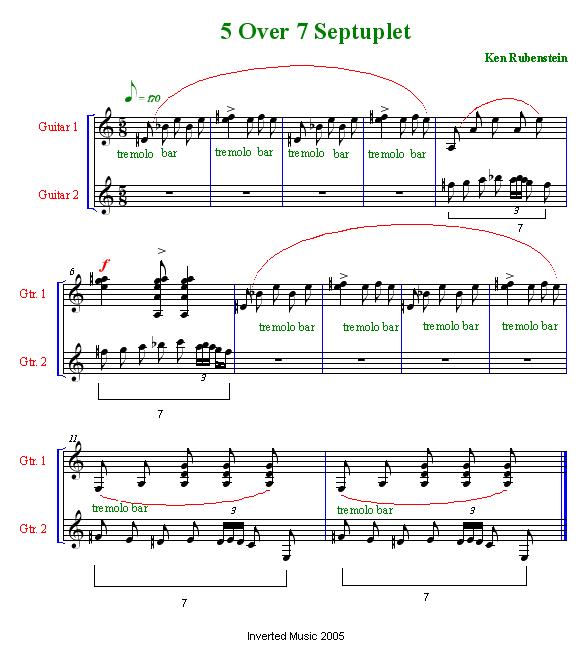
If you were to just attempt to feel a 5/7 beat, that would be hard. It would be hard in the context of truncation. You would be speeding up and slowing down to try and fit it in just right. But, if you just program that click track and adjust tempos accordingly, you will have those seemingly inaccessible 5/7th beats spelled out for you. Then you rehearse it for a half hour and you nail the thing.

This is something that's been explored and applied before, just maybe not with these particular numbers and patterns. Still, you might find it fun to check out.